Hello and welcome back to Dr Toby! In today’s article, I will be discussing Just Cause 3, in particular, Rico’s amazing grapples, and the trouble they could cause if you got your hands on one. This (hopefully) multi-part series will examine Just Cause 3 from every angle, addressing every weird and peculiar bit of science found within.
Now the first question to ask regarding tethers, before any of the fun stuff like speeds or forces or counting frames, is how long are they? Now, for some strange reason, there’s no record of how long Rico’s tethers are, all results which Google would provide me with link to unlimited tether mods, something antithetical to the rigorous scientific examination going on here. So, diving into the game, I had a think of what would be the easiest way to measure the length of the tethers, and came upon pixel measurements!
Pixel measurement is a method of discerning the length of in-game items, by comparing them to other in-game items which you know the size of. Oftentimes you must count the relative lengths in pixels to get the highest accuracy, ergo the name pixel measurements. So, I found a nice flat bit of road, a small bridge in Sirrocco Sud, aimed as far away as my grappling hook would let me, and then laid out a tether on the ground, from the furthest point of the grapple to the ground beneath my feet. Then, I stole a car from a middle-aged man and placed it just behind the cable.
Using High-End-Photo-Editing-Software (Paint) I counted the pixels making up the length of the car and the cable, and compared them. Because I didn’t get a perfectly level photo the lengths were at an angle, necessitating the use of the school child’s bane, the Pythagorean theorem! On a 1080p monitor, the car, a “Stria Ghepardos 3S” (this is important) measured 71 by 1 px, which by squaring, adding together then square rooting, gave us a complete length of 71 pixels (a very shallow angle). Meanwhile, the cable, being much larger measured 1369 by 40 pixels.
Let’s do this one together shall we?
a^2 =b^2 + c^2
a^2=1369^2 +40^
a^2=1,874,161+1600
a=sq(1,875,761)
a=1369.5

So as we can see, it’s still a very shallow angle (thank goodness) and so it’s not much bigger either. Dividing the cable length by the car length we find that the cable is equal to 19.28 car lengths, which isn’t much use until we remember that most of the cars in Just Cause 3 are modeled off of real-world cars and therefore most likely have a length we can work out, giving us the length of the cable!
According to the Just Cause Wikia, the Stria Ghepardos 3S most resembles a car by the name of the Alfa Romeo 8C Competizione, which looks very similar to our car, and has a length of 4.381m. Timesing our figure of 19.28 by the length of our car, we find that the length of the grappling cable is 84.46, which we shall round to 84.5 meters for simplicity — and, to acknowledge the futility of getting accurate measurements on this scale anyway.
Next up we need to work out the acceleration and speeds reached by Rico and his grappling hook over the course of a grapple. By recording footage of Rico grappling around and playing it back in slow motion I was able to get several timecodes representing Rico and the grapple’s trajectory. Included below is a table of all relevant points in the action.
| Event | Timecode (this is all half-speed) |
| Grappling hook fired | 0 |
| Grappling hook lands and Rico takes off | 1.224 |
| Rico stops accelerating (6m of accelerating) | 4.724 |
| Rico starts slowing down (0.2m of deceleration) | 10.474 |
| Rico stops | 11.723 |
Now for the really fun part. To work out the speed and acceleration Rico attains while grappling along. The easiest bit, and the one we’re gonna do first, is Rico’s speed in the middle of the grapple when he is moving at a constant speed. The formula used for calculating speed is very simple, speed is equal to the displacement divided by the time taken. S=d/t.
As we worked out earlier that the longest distance Rico can grapple in Just Cause 3 is 84.5 meters/ 6.2 meters of that is Rico accelerating and decelerating, so 78.3 meters of that is the middle, stable part. Looking back at our table we can see that this period is 2.875 seconds long ((10.474-4.724)/2) and therefore, the speed is equal to.
S=78.3/2.875
s=27.2ms^-1
27.2 meters per second.
That’s a lot of speed, 98 kilometres per hour, or the speed of a car on the motorway. This is only the start though, with the middle speed we can now work out the acceleration of the start and end phases.
The formula for acceleration is a=(dv)/dt
So, we know that Rico starts off stationary (relative to the Earth at least) and ends up travelling at 27.2 meters per seconds, and he achieves that change in velocity in 1.75 seconds.
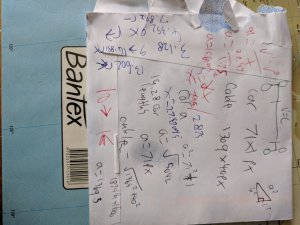
a=27.2/1.75
a=15.5ms^-2
And for the ending section, Rico decelerates down from 27.2 meters per second to 0 meters per second in 0.625 seconds.
a=-27.2/0.625
a=-43.52ms^-2
Let’s look at those figures then and see what we can get for them.
To make them more workable we’ll convert them to gees, a measure acceleration tied to the gravitational force of the Earth. At sea level, the Earth pulls you down at 9.807 meters per second squared, or one g.
Therefore in Just Cause 3, Rico accelerates at 1.5 gees (15.5/9.807) and decelerates at 4.4 gees (42.52/9.807). 1.5 gees thankfully isn’t that much, it’s fairly easily attainable by redlining a high-end consumer car — its fast, but not crazy fast.

Sadly for Rico though, in a car you’re supported by a nice fancy seat which keeps you in a position suitable for high gees, and generally keeping you all in one piece. Rico doesn’t have that luxury, he’s attached to that force by a rope attached to his forearm. What’s worse, his body is orthogonal (at right angles) to the force, which is going to make it much worse.
Literature on the force required to dismantle the human body is sorely lacking, but a combination of anecdotal evidence and the limited reports on the subject indicates that in all likeliness, Rico is spraining his shoulder or in the worst case, especially considering how often he grapples around, dislocating it.
For the decelerating phase, things get a little more interesting. Interestingly 4.2 gees is the world record for drag cars, and is just shy of the force which pilots could pull in WWI during dogfights. Of particular note, in this case, is Rico’s posture inJust Cause as he slows down. Instead of using a parachute, air brakes or just air friction to slow down, Rico uses his leg to brake to a stop, angling up to then stand on that leg. In all likelihood, that leg is broken, which we can check!
Articles on the acceleration needed to break bones are sorely lacking, but articles quoting force are plentiful! To work out the force we need to use Newton’s first law of motion, f=ma.
According to the official Just Cause wiki, Rico is 81 kilos, and assuming he’s carrying his guns and equipment we can assume his total mass is something closer to 100 kilograms. The acceleration we worked out before so it’s just a simple multiplication of 100*43.52 to end up with:
f=100*43.52
f=4352N
f=4.3kN
As I mentioned before literature on the force required to break bones is plentiful, and various sources provide the breaking point of a leg bone at around four thousand newtons, a fair bit less than the 4.3 thousand newtons we’re playing with. So yeah, broken leg.
Hopefully, Rico can survive this encounter with the ground with his limbs all attached, but it’ll be iffy. For a comparison, go to your local motorway, get up to a hundred kilometers an hour, then open the car door and try to stand up on the road. (*Please Don’t Actually Try This Kids*)
If he’s lucky, your friendly neighbourhood terrorist is going to end up with a broken and raw leg, as well as heavy scrapes on the rest of his body. And, since the accelerating force is going backwards and up through his body, a momentary redout as the blood in his body flows towards his head.

Bearing in mind for just a moment this is all predicated on the fact that Rico is travelling sideways (a common trend in Just Cause 3). Vertical travel is highly risky (ignoring for a moment the stupidity that is grappling towards the ground to slow yourself down) and nearing suicidal. Grappling upwards would entail constantly fighting against the everpresent one g of the earth, and probably resulting in a blackout as the grappling hook struggles to pull Rico up, sans blood in his head. Meanwhile grappling downwards would just accelerate past terminal velocity, most likely resulting in a loss of consciousness from g-forces (GLOC) and, well, a splat on the pavement when he tries to end his joy ride.
And that’s it for tethers today, while there are many more places we could go and much more we could experiment with, we have hit the limit of accuracy when dealing with video games. Sadly we cannot work out the composition that the grappling cable would require to not be torn to pieces, or just how much force two tethers could apply, or what would happen if two tethers were attached to the same object.
If you have another scientific gaming mystery you want us to examine, drop a comment below with your idea!
Please remember that this is all in jest, we very quickly went beyond our understanding of how any of this works. While all attempts to make this as accurate as possible were taken, there are of course going to be mistakes and misunderstandings of how some complex bit of physics works. The primary purpose of this article was fun with a bit of science mixed in. Please read in a similar frame of mind.
Comments are closed.